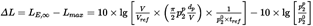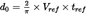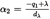- Latest available (Revised)
- Point in Time (31/01/2020)
- Original (As adopted by EU)
Commission Directive (EU) 2015/996Show full title
Commission Directive (EU) 2015/996 of 19 May 2015 establishing common noise assessment methods according to Directive 2002/49/EC of the European Parliament and of the Council (Text with EEA relevance)
You are here:
- Show Geographical Extent(e.g. England, Wales, Scotland and Northern Ireland)
- Show Timeline of Changes
More Resources
When the UK left the EU, legislation.gov.uk published EU legislation that had been published by the EU up to IP completion day (31 December 2020 11.00 p.m.). On legislation.gov.uk, these items of legislation are kept up-to-date with any amendments made by the UK since then.
This item of legislation originated from the EU
Legislation.gov.uk publishes the UK version. EUR-Lex publishes the EU version. The EU Exit Web Archive holds a snapshot of EUR-Lex’s version from IP completion day (31 December 2020 11.00 p.m.).
Changes over time for: Division E3
Alternative versions:
Status:
EU Directives are published on this site to aid cross referencing from UK legislation. Since IP completion day (31 December 2020 11.00 p.m.) no amendments have been applied to this version.
E3CONSISTENCY OF MAXIMUM AND TIME INTEGRATED METRICS — THE SCALED DISTANCEU.K.
A consequence of using the simple dipole model to define the energy fraction is that it implies a specific theoretical difference ΔL between the event noise levels Lmax and LE . If the contour model is to be internally consistent, this needs to equal the difference of the values determined from the NPD curves. A problem is that the NPD data are derived from actual aircraft noise measurements — which do not necessarily accord with the simple theory. The theory therefore needs an added element of flexibility. But in principal the variables α1 and α2 are determined by geometry and aircraft speed — thus leaving no further degrees of freedom. A solution is provided by the concept of a scaled distance dλ as follows.
The exposure level LE,∞ as tabulated as a function of dp in the ANP database for a reference speed Vref, can be expressed as
where p 0 is a standard reference pressure and tref is a reference time (= 1 s for SEL). For the actual speed V it becomes
Similarly the maximum event level Lmax can be written
For the dipole source, using equations E-8, E-11 and E-12, noting that (from equations E-2 and E-8) 
This can only be equated to the value of ΔL determined from the NPD data if the slant distance dp used to calculate the energy fraction is substituted by a scaled distance dλ given by
or
Replacing dp by dλ in equation E-5 and using the definition q = Vτ from Figure E-1 the parameters α1 and α2 in equation E-9 can be written (putting q = q1 at the start-point and q – λ = q2 at the endpoint of a flight path segment of length λ) as
Having to replace the slant actual distance by scaled distance diminishes the simplicity of the fourth-power 90 degree dipole model. But as it is effectively calibrated in situ using data derived from measurements, the energy fraction algorithm can be regarded as semi-empirical rather than a pure theoretical.
Options/Help
Print Options
PrintThe Whole Directive
PrintThe Whole Annex
PrintThis Division only
You have chosen to open the Whole Directive
The Whole Directive you have selected contains over 200 provisions and might take some time to download. You may also experience some issues with your browser, such as an alert box that a script is taking a long time to run.
Would you like to continue?
You have chosen to open Schedules only
The Schedules you have selected contains over 200 provisions and might take some time to download. You may also experience some issues with your browser, such as an alert box that a script is taking a long time to run.
Would you like to continue?
Legislation is available in different versions:
Latest Available (revised):The latest available updated version of the legislation incorporating changes made by subsequent legislation and applied by our editorial team. Changes we have not yet applied to the text, can be found in the ‘Changes to Legislation’ area.
Original (As adopted by EU): The original version of the legislation as it stood when it was first adopted in the EU. No changes have been applied to the text.
Point in Time: This becomes available after navigating to view revised legislation as it stood at a certain point in time via Advanced Features > Show Timeline of Changes or via a point in time advanced search.
See additional information alongside the content
Geographical Extent: Indicates the geographical area that this provision applies to. For further information see ‘Frequently Asked Questions’.
Show Timeline of Changes: See how this legislation has or could change over time. Turning this feature on will show extra navigation options to go to these specific points in time. Return to the latest available version by using the controls above in the What Version box.
More Resources
Access essential accompanying documents and information for this legislation item from this tab. Dependent on the legislation item being viewed this may include:
- the original print PDF of the as adopted version that was used for the EU Official Journal
- lists of changes made by and/or affecting this legislation item
- all formats of all associated documents
- correction slips
- links to related legislation and further information resources
Timeline of Changes
This timeline shows the different versions taken from EUR-Lex before exit day and during the implementation period as well as any subsequent versions created after the implementation period as a result of changes made by UK legislation.
The dates for the EU versions are taken from the document dates on EUR-Lex and may not always coincide with when the changes came into force for the document.
For any versions created after the implementation period as a result of changes made by UK legislation the date will coincide with the earliest date on which the change (e.g an insertion, a repeal or a substitution) that was applied came into force. For further information see our guide to revised legislation on Understanding Legislation.
More Resources
Use this menu to access essential accompanying documents and information for this legislation item. Dependent on the legislation item being viewed this may include:
- the original print PDF of the as adopted version that was used for the print copy
- correction slips
Click 'View More' or select 'More Resources' tab for additional information including:
- lists of changes made by and/or affecting this legislation item
- confers power and blanket amendment details
- all formats of all associated documents
- links to related legislation and further information resources